Home	PREFACE	PRIMEVAL	SPECIAL	GENERAL	CONCLUSION
		\|
Dictionary	Events	Aristotle	Galileo	Newton

Aristotle's Spacetime

Space and Time according to Aristotle
Spacetime according to Aristotle
Aristotle and Absolute Rest
Aristotle's Laws of Physics


Aristotle (384-322 B.C.)

Space and Time according to Aristotle

Every sensible body is by its nature somewhere. (Physics,Book 3, 205a:10)
Time is the numeration of continuous movement. (Physics, Book 4, 223b:1)

Aristotle was interested in motion. He realized that motion can be understood by seeing how the location of an object changed. And that one could talk about "one object moving faster than another" by comparing how much the location of each changed in some interval of time.

It is not our purpose to critically discuss Aristotle and his views concerning space and time. We are exercising our poetic license to use him to represent the ancient pre-Renaissant views of space and time.

Spacetime according to Aristotle

(Poetic License, Technical comments)

Let us a try to draw a picture of Aristotle's view of space, time, and motion.

Recall: We are trying to understand the physical relationship between events. We do so by modeling the set of events with a mathematical model: spacetime. A good model should accurately display certain features that reflect these relationships. For spacetime-models, these features are certain geometric figures that are somehow automatically built into the model.

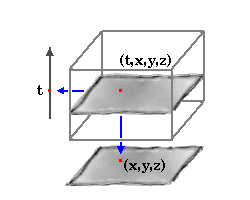
Imagine a 4-dimensional cube, sliced into a stack of horizontal 3-planes.
(If this bothers you, revisit the discussion on 4-dimensionality in Introducing Spacetime. Don't forget to come back using !)


ARISTOTLE'S SPACETIME Each horizontal plane represents "an instant of time". Each point on that plane represents "a position in space (at that instant of time)".

Here are some key features of this model:

We have a 4-dimensional model because events require 4 numbers to label them.
We use certain rigid mathematical structures to reflect certain "absolute" or "universal" physical notions.
- In general, there are many 3-planes that can be picked out of our 4-dimensional cube. However, because we have sliced up our cube in a certain way, there is a special set of planes: namely, the HORIZONTAL PLANES. Each horizontal plane represents "an instant of time". More precisely, it represents "all of space at an instant of time". In other words, each horizontal 3-plane consists of those events which are simultaneous to each other.
- Since we started with a cube, there is also special set of lines: namely, the VERTICAL LINES, since they are parallel to the vertical sides of the cube, as well as perpendicular to the horizontal planes. Each vertical line represents "a position in space". More precisely, it represents "for all of time, it is the same position in space". In other words, each vertical line consists of those events which are coincident in space.
- The TIME of an event is recovered by "finding the shadow" of the event (by following the side-pointing blue arrow) onto the vertical t-axis.
- The LOCATION IN SPACE of an event is recovered by "finding the shadow" of the event (by following the downward-pointing blue arrow) onto the horizontal plane.

Aristotelian Map Reading 101:
How to recover "space" and "time" from "spacetime"

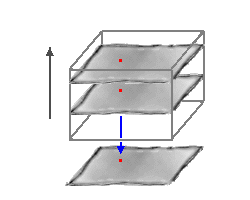
Two events at the same place: "coincident in space"
(...since these two events lie on the same vertical line.)

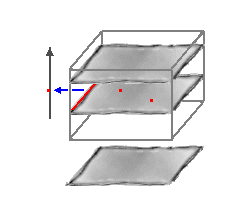
Two events at the same time: "coincident in time" or "simultaneous".
(...since these two events lie on the same horizontal plane.)

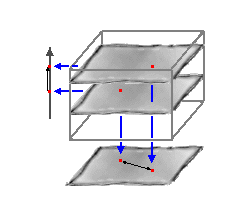
The "time elapsed" (temporal separation) between two events.
(Find the shadows of these events onto the vertical line, then measure this time-difference by measuring the length along the vertical line.)
The "spatial distance" (spatial separation) between two events.
(Find the shadows of these events onto a common horizontal plane, then measure this distance on this horizontal plane.)

This then will be our first spacetime model, that is, our first mathematical model of the physical notion of "the history of the universe".

And, the Aristotelians have provided us a method by which we can recover "space" and recover "time" from "spacetime".

Given a set of events for the whole universe:
slice it up into a rigid stack 3-planes (to decide what we call "time" and what we call "space").
make a "space" measurement of a particular event ("how far it is away from me") by looking "on which vertical line" the event sits (which is intuitively "finding the shadow it casts on a horizontal plane").
make a "time" measurement of a particular event ("how long ago" or "how long to wait") by looking "on which horizontal plane" the event sits (which is intuitively "finding the shadow it casts on a vertical line").

This is essentially (but not exactly) the procedure to recover "space" and "time" from any model for "spacetime". The basic idea is here. But we will see that we will need to refine the details.

Aristotle and Absolute Rest

Aristotle believed that "being at rest" was the natural state of motion of any object. If an object were in motion, then there must be some agent that is responsible for that motion. And when that agent stops, the motion stops.

According to Aristotle, there is a privileged being: The Prime Mover. He is the first agent, responsible for moving objects, which, in turn, move other objects.

The Prime Mover, he argued, must be at Absolute Rest. By "absolute" rest , we mean that all observers will universally agree on that state of rest.

In this spacetime, The Prime Mover has a vertical worldline since he is always at rest. That is to say, he is always in the same position at each instant in time.

A poetic interpretation of Aristotle's Spacetime

One can think of Aristotle's Spacetime in the following way.

The Prime Mover carries a big clock on his chest. He has a gridwork (or "jungle-jim") of metersticks laid out everywhere in space. With a loud voice, which can be heard everywhere instanteously, he declares "Take a branding-iron and sear each point in space with three numbers (x,y,z) that locate how far in the x, y, and z directions that point is from Me, who sits at x=0, y=0, z=0."

This is Aristotle's Spacetime. To recover space, look at the label branded on that point in space. To recover time, listen to the Prime Mover's clock announcements.

Aristotle's Laws of Physics
(although incorrect)

For completeness, we mention some of what could be called Aristotle's Law of Physics.

ARISTOTLE'S LAW OF INERTIA
A body, not acted on by any force, remains at absolute rest.

ARISTOTLE'S LAW OF MOTION
If, then, A is the moving agent, B the mobile, C the distance traversed and D the time taken, then A will move B/2 over the distance 2C in time D, and A will move B/2 over the distance C in time D/2; for so the proportion will be observed. (Physics VII 249b-250a)
Force is proportional to the velocity. F=mv.

ARISTOTLE'S LAW OF GRAVITATION
Heavy objects fall faster than light objects.

We will eventually see that physical experiment shows that all of these statements are actually FALSE.

Home		PREFACE	PRIMEVAL	SPECIAL	GENERAL	CONCLUSION
Dictionary		Events	Aristotle	Galileo	Newton

Rob Salgado
27-Nov-1995