Home PREFACE PRIMEVAL SPECIAL GENERAL CONCLUSION
Dictionary Newton Equivalence Einstein Schwarzschild

Introducing The Einstein Principle of Equivalence

(Technical comments)

Einstein was looking to extend his Principle of Relativity to more general siutations. In Special Relativity, no physical experiment could distinguish one state of uniform velocity from another. Could this be extended to "no physical experiment could distinguish free motion"?

How does gravity fit into this?

Galileo's Principle of Equivalence: Inertial mass = Gravitational mass
In other words, given an event and an initial velocity vector, a particle will travel a definite curve (regardless of its mass or composition).

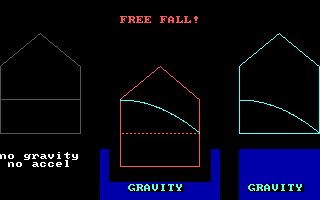Einstein's Principle of Equivalence:

Einstein extended Galileo's Principle of Relativity.

The Equivalence Principle: Free Fall in a Gravitational Field
(144 kb)
(24 kb)

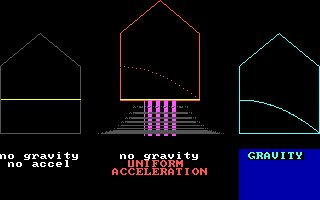The Equivalence Principle with light rays
(160 kb)
(31 kb)
This led Einstein to predict that light is bent by the gravitational field around the Sun.

The Deflection of Starlight

In this spacetime diagram, the Sun is at rest. The earth at one instant (the blue dot) is observing a light ray (the red curve) that came from a distant star. Because of the influence of the gravitational field near the Sun (the yellow tube), the light ray was deflected as it passed near the sun. To the earth, that star appears to be in the direction of the dotted-black line (since we "see" by tracing back along the incident direction). However, the actual trajectory in space is along the dotted-red curve.

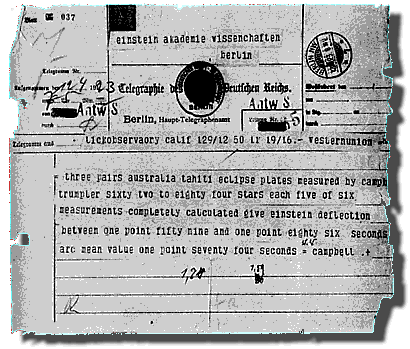
One prediction that Einstein deduced his theory is that starlight will have an angular deflection of 1.75 seconds of arc.

Such an effect was observed in 1919. Astronomers waited until for a good solar eclipse, in order to get a better view of starlight grazing the Sun. The stars appeared shifted from their true positions, which had been obstructed (following a straight-line path) by the Sun.


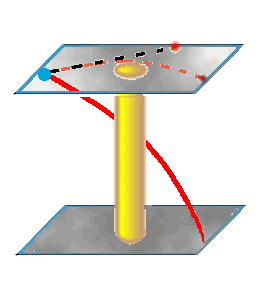
Success in a subsequent measurement in 1922.

Recall that light rays "ride" on the Light Cones. If the Light Cones were arranged uniformly, then light would travel in straight lines in spacetime.

But this physical experiment demonstrates that the Light Cones cannot be arranged uniformly in spacetime: spacetime must be curved.

Home PREFACE PRIMEVAL SPECIAL GENERAL CONCLUSION
Dictionary Newton Equivalence Einstein Schwarzschild
Introducing CURVATURE: Einstein-Riemann Spacetime